William Marks
As of this writing, the Miami Heat have won 22 consecutive games. Meanwhile over in the NHL, from January 19th through March 6th, the Chicago Blackhawks earned at least one point in 24 consecutive games. While the Heat’s streak is about the same length, the Blackhawks’ run appears more impressive, given that at the time, they had earned a point in every game they had played this season. (Points are earned in a win (2 points) or an overtime loss (1 point)) To determine which streak was actually more difficult to pull off, I looked at the money lines of each team’s games over the length of their respective streaks. I then converted the money line from each game into the odds of winning. Under the assumption that each game is an independent event, I calculated the probability of the Heat winning all 22 games (according to Vegas odds) to be 0.2344174%. Then, treating each of the games in which the Blackhawks earned a point as a win, I found the probability of their streak to be 0.0000597915%. When it comes down to the odds, the streak the Blackhawks put together looks much more unlikely in hindsight, considering how infinitesimally small the probability of this points streak is compared to the Heat’s run. Given the relative randomness of hockey, the Heat would have to extend their current streak by a significant margin to even approach a streak as unlikely as the Blackhawks’.

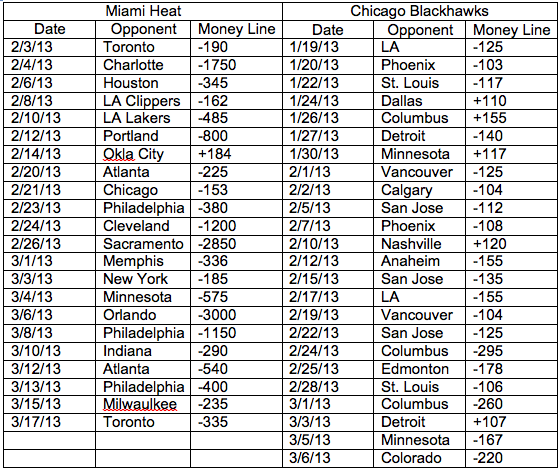
I feel as though the Blackhawks’ figure is inaccurate as an overtime loss would be a loss for a bettor. Wouldn’t the moneyline on a “win or overtime loss” be more appropriate in these instances?
Since the game-by-game probabilities are a function of how relatively good the Heat and Blackhawks are/were, then “impressive” is from the standpoint of probability. But isn’t it impressive also that the Heat were good enough to make the probability of winning 22 straight games that high?
I like your approach and I agree with Dan’s point. Just wondering, when you converted moneylines did you take into account the vig? For example did you take -110 as a 50% chance?