By David Roher
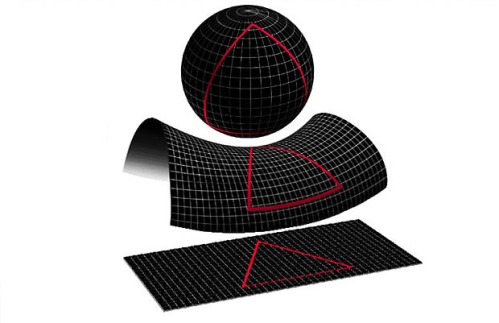
One of the great cosmological questions has to do with the shape of our universe. There are three possibilities: spherical (positively curved), hyperbolic (negatively curved), and flat (no curve). According to the latest data (see the link below the picture for more details), it’s probably flat. Which is probably good, because all kinds of crazy stuff would happen if it weren’t. In a spherical universe, if you took off on a rocket ship and never changed direction, you’d eventually get back to where you started. In the saddle-shaped universe, parallel lines would diverge from each other.
As a history major, I’ll stop the astronomy interlude here before I get even further over my head (although I do think wormholes would be a plausible explanation for some behavior in the sports world). The reason I bring it up? If you said payroll efficiency, you win the Poorly Constructed, Overly Drawn-Out Metaphor Interpretation Award!
As part of a larger look at salary, efficiency, and competitive balance, I was thinking this morning about the relationship between a player’s salary and production. In general, the more someone produces, the higher the compensation. But this inevitably doesn’t work out, so spending efficiently becomes one of the most important jobs of a front office. What I was wondering this morning is how we can expand the above salary-production statement. Basically, I want to explain how salary relates to not only production, but also efficiency.
I see three possibilities, and they all have to do with curvature.
- The first is that as salary increases, player production increases at a proportionally faster rate than salary. In other words, as salary increases, efficiency increases.
- The second is that as salary increases, player production increases at a proportionally slower rate than salary. In other words, as salary increases, efficiency decreases.
- The third is that as salary increases, player production increases at a rate proportionally equal to salary. In other words, as salary increases, efficiency remains constant.
Mathematically inclined people might realize that I’m just talking about the second derivative being positive or negative. But please indulge me – I like outer space more than math. The shape of the universe is quantified by the variable Ω. In a spherical, positive-curvature universe, Ω is greater than 1. In a hyperbolic, negative-curvature universe, Ω is less than 1. And in a flat, no-curvature universe, Ω is equal to 1. Let’s take a look at each of these possibilities.
The Positively Curved Sports Universe (Ω>1)
League in which this Universe might exist: The NBA. As a result of a high minimum (over $1 million for players with 5 or more years experience) and a low ceiling (LeBron James probably won’t be allowed to make over $17 million per year in the first couple years of his next deal), there’s a good chance that the NBA’s salary system might actually result in a scenario where highly paid players are more deserving of their salary than average or below-average players. There’s a chance that fixed low salaries coming out of the draft might drag omega down, but if someone did a study, my guess is that s/he would find this result.
The Negatively Curved Sports Universe (Ω < 1)
 League in which this Universe might exist: Major League Baseball. In MLB, players must have at least two or three years of experience before they go to arbitration, and at least six years of experience before they reach free agency. As a result, most players have their career year before teams can pay them as much as they would actually get for that year on the free market. Even when they do become free agents, the lack of any upper bound results in players often receiving impossibly large deals that would be a stretch to live up to even if they performed according to their projected value (and they very often don’t), even if one allowed for the increased degree to which these veterans drive ticket sales. I’m extremely confident that a study here (which I’m planning on doing) would result in an omega less than one.
League in which this Universe might exist: Major League Baseball. In MLB, players must have at least two or three years of experience before they go to arbitration, and at least six years of experience before they reach free agency. As a result, most players have their career year before teams can pay them as much as they would actually get for that year on the free market. Even when they do become free agents, the lack of any upper bound results in players often receiving impossibly large deals that would be a stretch to live up to even if they performed according to their projected value (and they very often don’t), even if one allowed for the increased degree to which these veterans drive ticket sales. I’m extremely confident that a study here (which I’m planning on doing) would result in an omega less than one.
The Zero-Curve Sports Universe (Ω = 1)
League in which this Universe might exist: The English Premier League. The Premier League has almost no restriction on any type of contract a team can offer any player. Given that and the amount of scrutiny that goes into acquisitions, the efficiency is likely unchanged for any salary. However, the occasional bust of a well-paid player or breakout or a low-paid player could drag omega a little bit below 1. I don’t know enough about the league to know how close it would be.
Which of these shapes do you think is best for fans? A flat Universe is a result of free-market spending that interferes with competitive balance. But one that is too curved has other problems. In any case, I hope that thinking about salary efficiency in this way provides a framework for future work.



I would think the flat universe would be best. Paying for actual production would get rid of distortions, especially in baseball, where teams end up paying for past perfromance.
As a longtime follower of English soccer, I think your example for the flat universe is a little off. Because soccer is a sport in which player evaluation is very difficult due to the lack of useful stats, players are often bought on the basis on one or two good seasons. Contracts are guaranteed so there is no incentive for an underperforming player to move if they have to take a pay cut. In addition, soccer players are bought and sold (IE teams pay each other for a player’s rights) so the market is distorted. A top player might cost a team £25m before salary. As a result free agents can demand proportionately higher salaries.
To achive a ‘flat universe’ there would need to be nearly perfect methods of player evaluation and a free labour market which would prevent overvalued players setting benchmark salaries. Basketball seems to have high evaluation success rates (at least at the top) and the NFL has a high level of player mobility because contracts are not guaranteed.
Perhaps a better measure than pure salary would be some measure of the total cost a player is to a team, ie annual salary plus (transfer fee/years of contract), which starts sounding very similar to the salary cap numbers from the NFL.
Another thing which I think will influence omega is that the owners of the biggest spending clubs don’t necessarily appear to be “rational” in their decisions – the most obvious case of this recently is Manchester City, who were taken over by a Middle Eastern consortium, and went and spent _lots_ of money, probably overpaying for players along the way.